A response to Sasha Gusev on IQ
The weight of evidence suggests genes matter far more than family environment.
Written by Noah Carl.
Has the heritability of IQ been substantially overestimated due to lack of controls for environmental confounding? That’s the message of two recent articles by Sasha Gusev, an associate professor at the Harvard Medical School.
I should say at the outset that the articles are very much worth reading. With the exception of one or two snarky paragraphs, they are largely free of the churlish tone Gusev became known for on Twitter. And the evidence they present is actually compelling. It’s not just the usual: “twin studies… something something… EUGENICS.” However, this doesn’t mean I’m persuaded by all or even most of the author’s claims.
And some of his claims are rather sweeping indeed. He says that molecular genetics has “thoroughly debunked” the view that “intelligence is just like any other biological trait, with individual differences explained by simple genetic causes that are easily quantifiable and culturally immutable”. (Okay, individual differences in IQ aren’t culturally “immutable”, but we knew that long before molecular genetics came along.) He also says that a trait like IQ “appears to be highly culturally and environmentally dependent” – not just culturally and environmentally dependent but highly so.1
The focus of Gusev’s first article is a handful of recent studies in molecular genetics which he argues point to much lower heritability than previously believed, as well as a major role for “cultural forces” in shaping IQ. The focus of his second article is addressing comments on the first, especially in relation to twin studies, which he argues overstate heritability due to the erroneous “equal environments assumption”. This is the assumption that identical twins reared in the same household do not have more similar environments than non-identical twins reared in the same households (as regards things like parenting, education and other potential causes of IQ). The assumption would be false if, say, people tend to treat identical twins more similarly than non-identical twins and this affects their IQs.
In the present article, I will not comment on the molecular genetic studies Gusev cites – in part because I am not well-versed in the ins and outs of the relevant methods, and in part because Joseph Bronski has already addressed Gusev’s interpretation of them. Rather, I will argue that those studies should not cause us to update our priors very much (not nearly as much as Gusev claims) because they have to be weighed against all the evidence from behaviour genetics, not just twin studies.
To begin with, the equal environments assumption isn’t something behaviour geneticists have just blindly accepted without ever bothering to test it. There is a voluminous literature on the “EEA”, which seems to suggest that while the assumption is not strictly true, violations of it are unlikely to substantially change heritability estimates. I won’t bother going through the entire literature, but I do want to highlight two quite elegant research designs that support the EEA’s validity.
The first involves comparing misclassified twins (i.e., those who are mistaken about their zygosity). In a 2013 study, Dalton Conley and colleagues analysed data from the US, and found that pairs of identical twins who knew they were identical were about as similar with respect to GPA as pairs who incorrectly believed they were not identical.2 The second involves comparing unrelated lookalikes (individuals that happen to look very similar but are completely unrelated). In a series of studies, Nancy Segal and colleagues have given personality tests to pairs of such individuals (most of whom were originally identified by a Canadian photographer for a specific project). Unsurprisingly, the pairs turned out to be no more similar than expected by chance.3
The chart below, taken from a paper by David Cesarini and Peter Visscher, shows correlations for different types of brothers in Sweden. With the exception of years of schooling, the traits were measured at age 18. Because there were at least 600 pairs in each category, all the correlations are estimated precisely (there was no need to add confidence intervals).
Focus on the orange squares, corresponding to “cognitive skills”, and compare monozygotic twins to adoptees. Both types of brothers were raised in the same household, but one type shares all their genes and the other type shares none. What do we see? Monozygotic twins are vastly more similar than adoptees. In fact, there is a clear association between genetic relatedness and trait similarity: half-siblings are more similar than adoptees, full siblings are more similar than half siblings, and monozygotic twins are more similar than full siblings. The most straightforward interpretation of this pattern is that sharing genes makes people similar because genes affect traits.
Now, it is theoretically possible that the main reason monozygotic twins are so much more alike than adoptees (despite both types being raised in the same household) is that people treat monozygotic twins more similarly. However, this just isn’t very plausible. As noted above, the equal environments assumption has been tested extensively and violations of it do not seem to be a serious issue.
What’s more, even quite intensive interventions have been unable to boost IQ in the long run because of something called “fadeout”. In a 2015 study, John Protzko meta-analysed 39 RCTs of interventions designed to boost IQ and found that the average impact declined (gradually) to zero after the interventions ended. Some quasi-experimental research suggests that education boosts IQ even in the long run. Yet other research suggests it doesn’t affect intelligence per se, or only does so to a very limited extent.
I should note that the pattern in the chart above is also consistent with environmental effects on IQ. Holding genetic relatedness constant, siblings raised in the same household are somewhat more similar than those raised apart, and adoptees are more similar than expected by chance. (Under a purely genetic model, the correlation between unrelated brothers would be zero.)
Which brings me to adoption studies. These are particularly useful for teasing apart the effects of genes and family environment since they do not make the “strong” assumption that monozygotic and dizygotic twins raised in the same household have equally similar environments.4 While the adoption literature as a whole suggests that both genes and family environment play a role in IQ, most studies find that genes are far more important.
The most widely touted study showing an impact of the family environment is a 2015 paper by Kenneth Kendler and colleagues. Exploiting a large Swedish sample, the authors compared individuals who had been adopted by more-advantaged families to their biological siblings who had not been adopted. They found that the former group scored 4.4 points higher on an IQ test taken at age 18, which provides fairly strong evidence that at least one intervention, namely adoption, can meaningfully raise IQ.5
However, other adoption studies suggest that any long-term impact of family environment is small compared to that of genes. In 1978, Sandra Scarr and Richard Weinberg examined to what extent adoptees’ IQs (measured in adolescence) were related to the IQs and other characteristics of their adoptive parents. They found that the correlations were extremely weak – much weaker than the correlations in a comparable sample of biological families. The authors also knew the education level of the adopted children’s birth mothers, and they found that this variable was more strongly related to the adopted children’s IQs than any variable corresponding to the adoptive parents. In other words, adopted children were more similar to their birth mothers than to the parents that had actually raised them.
Numerous subsequent studies have replicated this finding. In a 1997 paper, Robert Plomin and colleagues found that the correlation between adopted children’s IQs and the IQs of their biological parents increased between the ages of 4 and 16. In other words, adopted children became more similar to their biological parents as they got older. Meanwhile, the correlation between adopted children’s IQs and the IQs of their adoptive parents remained close to zero throughout childhood and adolescence.
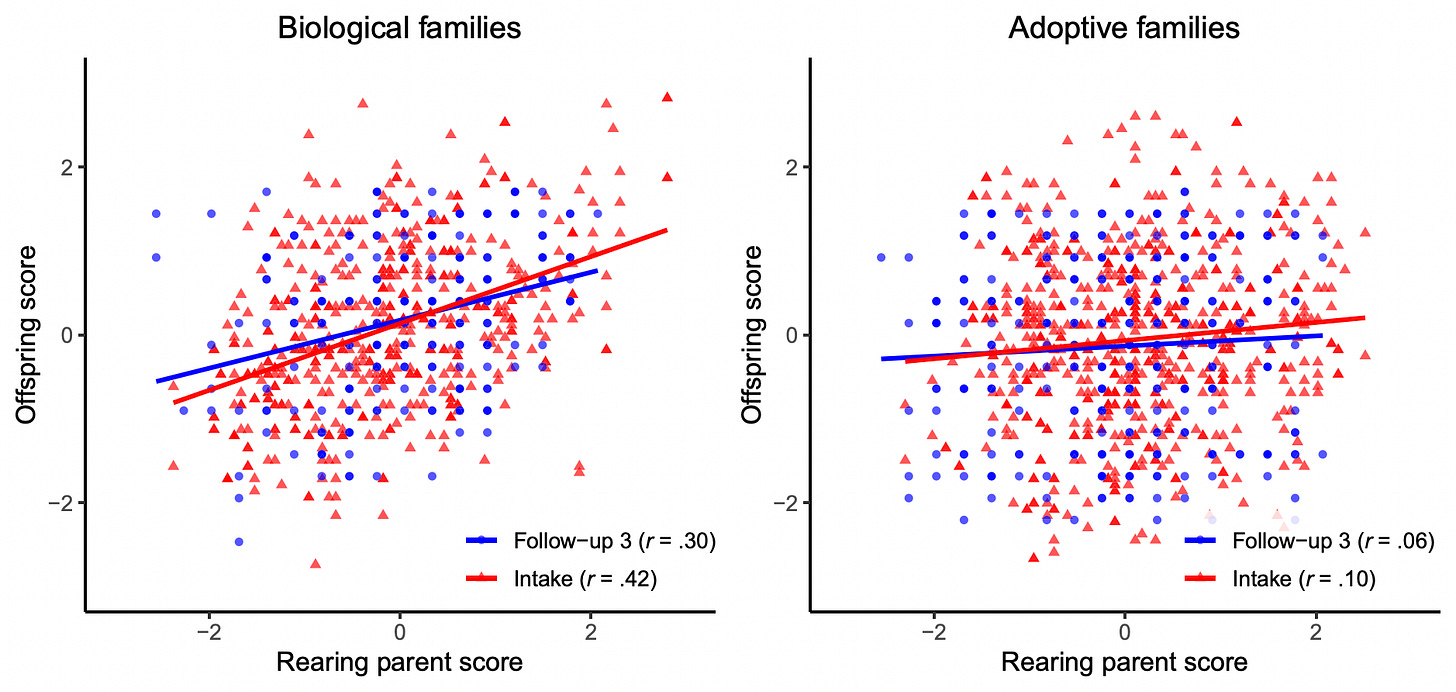
The latest replication of the Scarr and Weinberg result is a 2021 study by Emily Willoughby and colleagues. Their main finding is shown below: the lefthand chart plots the relationship between parents’ and children’s IQs in a sample of biological families, while the righthand chart plots the same relationship in a comparable sample of adoptive families. By age 30 (when the third follow-up took place) the adopted children barely resembled their adoptive parents at all. Note that because most of the adoptees were international placements, little information was available about their birth parents.
It is possible that correlations between adopted children and their adoptive parents are somewhat attenuated due to range restriction. Adoptive families tend to be positively selected, so they will not reflect the full range of family environments in the population. However, this cannot account for the fact that adopted children are more similar to their biological parents than to their adoptive parents, since there is also range restriction among the families from which adopted children come.
Another powerful adoption design involves comparing pairs of identical twins who were separated at birth and then raised in different families. This design does require the assumption that such individuals are not treated more similarly than two unrelated children would be. But as we have seen, studies of unrelated lookalikes show that people who look similar do not end up with similar personalities. Remarkably, identical twins reared apart turn out to be very similar in terms of IQ – almost as similar as identical twins reared together. Indeed, the average IQ difference between identical twins reared apart is close to the average difference between two testings of the same individual, as Thomas Bouchard and colleagues showed in their 1990 paper.
Nancy Segal and colleagues have extended this line of research by comparing identical twins reared apart to what they call “virtual twins” (unrelated siblings of the same age). They find that identical twins reared apart become more similar as they get older, while virtual twins become less similar. Not only that, but identical twins reared apart are much more similar than virtual twins. So people who share 100% of their genes but were raised in different households are much more similar than people who share none of their genes but were raised in the same household.
It is difficult to explain all the various findings from the adoption literature unless genes matter far more than family environment when it comes to IQ. These findings are highly consistent with the evidence from twin studies, as well as the lack of long-run impact in RCTs. For example, it is hard to imagine what kind of bias or source of confounding could explain why biological relatives living apart become more similar over time. Or why adopted children turn out more similar to their birth parents than to their adoptive parents. Overall, Eric Turkheimer’s “Second Law” of behaviour genetics holds up well: “The effect of being raised in the same family is smaller than the effect of genes”.
Gusev deserves credit for highlighting some interesting and puzzling findings from molecular genetics, thereby underlining that there really is a “missing heritability problem”. And yes, those findings should shift our priors toward a lower estimate for the heritability of IQ (at least for the time being). However, they shouldn’t shift our priors nearly as much as Gusev claims. Nor do they justify saying that IQ is “highly culturally and environmentally dependent” within contemporary Western societies.6 Has the heritability of IQ been substantially overestimated? Almost certainly not.
Noah Carl is Editor at Aporia.
Consider supporting Aporia with a paid subscription:
You can also follow us on Twitter.
In his article, Gusev actually refers to “a trait like IQ/education”, thereby conflating two quite different things. Indeed, it has long been known that education is subject to less genetic and more shared environmental influence than IQ. This is for several reasons. First, education is a fairly blunt instrument: two people with the same “years of education” under their belt can have very different IQs. Second, education is influenced by traits other than IQ, notably “non-cognitive skills” – which may be more malleable. Third, education (but not IQ) is something that wealthy parents can easily buy for their dull children.
The same was not true in a Swedish dataset, though there were only 14 misclassified identical twins.
In one study, Segal and colleagues did find a significant positive correlation for openness on one personality inventory. However, the correlation for the same trait on another inventory was negative and non-significant. According to the authors, the significant correlation may have arisen because some of the participants had volunteered to take part and may therefore have been self-selected for openness. (In addition, the p-value was not corrected for multiple comparisons.)
Adoption studies that involve comparing adoptees and biological children raised in the same household do make the assumption that the two groups have equally similar environments.
The finding is consistent with a 2005 meta-analysis, which reported a positive impact of adoption on IQ.





It seems to me clear that the heritability estimates from twin studies are correct, and the estimates from GWAS molecular genetics much too low. Here's the argument:
1) Twin studies measure the effect of the whole genome at once. That means that a sample size of ~ 50 will give a fair result.
2) GWAS studies are attempting to identify each genetic variation that contributes to differences in intelligence, and thence measure the effect of each genetic variation, and add these up.
3) Intelligence is a hugely complex trait; necessarily, any recipe for intelligence thus has to include a huge amount of information. That can only be if it involves a huge number of genes. You can't encode large amounts of information in a small number of genes.
4) Hence, variations in intelligence likely involve thousands of genetic variations (let's say 3000) each having (on average) a ~ 1/3000th effect on the overall difference.
5) The sample size needed to detect small signals scales as the square of how small the signal is.
6) Hence, for GWAS studies to find all of the genetic contributions to intelligence you'd need a sample size of ~ 50 * (1/3000)^2. That is 500 million. That is, you'd need to sample 500 million people's genomes. Obviously that hasn't been done.
7) If your GWAS study samples 1000 genomes then you can only find genes that contribute more than sqrt(1/1000th) of the variation. That is, the genes making ~ 3% contributions (actually. it's worse than that, for decent accuracy you need to repeat ~50 times, so you need a much bigger sample)
8) And that's exactly what they find, a handful of genes that affect intelligence at the ~ 3% level.
9) And that would be fine, if intelligence were indeed a trait controlled by ~ 30 genes each having a ~3% effect. But it can't be. There's no way one can encode the necessary oodles of information in 30 genes or even 300. Necessarily, it's going to involve 1000s of genes.
10) And that's why GWAS estimates are way lower; their sample sizes are only big enough to find the tip of the iceberg -- the small number of genes that each have a substantial effect -- but completely miss the thousands of genes that each contribute a small amount.
Refutations welcome; am I missing something?
Great article, your response to Mr. Gusev was quite thorough. Nevertheless this quote that you stated in relation to the “missing heritability” is the main point of contention where we “shouldn’t shift our priors nearly as much as Gusev claims.” I’m not sure the response you laid out is convincing enough to not shift our priors much more. In regard to this, I believe we should at the very least be much more cautious and have a much more probabilistic approach as it relates to the estimates of heritability. There still seems to be much we don’t understand in that regards it seems!